How Many Squares Are on a Chessboard? We Counted

We've been asked this question thousands of times, "How many squares are on a chessboard? We've got the answer! Let's break it down, board by board and column by column.

For instance, there is one square of size 8×8 units.

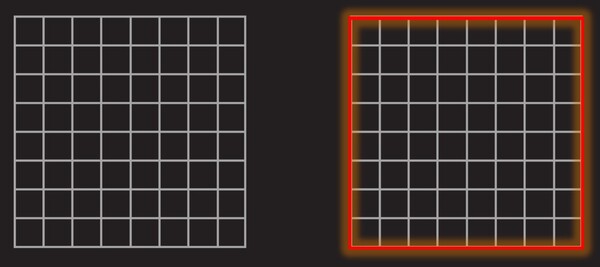
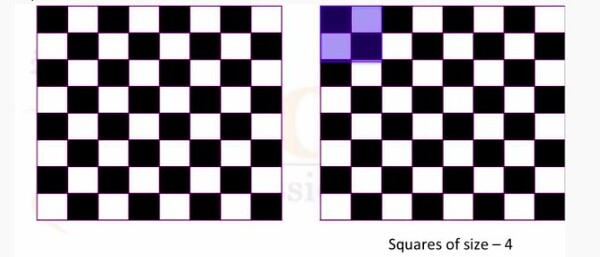
How many squares are on a chessboard? It has an answer that could not be any simpler: 64. Here's how we came up with this number! The board can be broken down into smaller squares, like the one below. If you break the 8×8 square into two 4×4 squares, there will be two squares of size 4×4 units. If you do this to every court on the board, there will be 8 x 4 = 32 total yards of size 4×4 teams. If you do this to every square on the board again (breaking it down twice), there would be 16 x 2 = 32 total yards of size 2x2 units.

Then there are four squares of size 7 x 7 units.

There are seven rows and seven columns on a 7 x 7 chessboard. Any number raised to an exponent is just the product of multiplying the number by the exponent. In other words, growing 10 to the power of 3 equals 1000 because 10 * 10 * 10 = 1000. Ten to the power of 4 equals 10000 because we're just multiplying ten by itself four times, so 10*10*10*10=10000.
Range of (7 X 7) squares = (7 - 7 + 1) * (7 - 7 + 1) = (1 * 1) = (1^2)
For that reason, the overall quantity of squares on a 7 x 7 chessboard = (1^2) + (2^2) + (three^2) + (four^2) + (5^2) + (6^2) + (7^2) = 7 * (7 + 1) * (2 * 7 + 1) / 6 = (7 * 8 * 15 / 6) = 140.

How many squares of size are 6 x 6 units

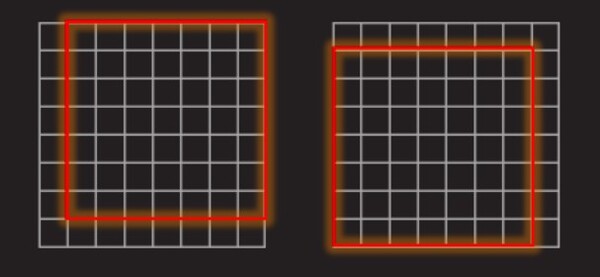
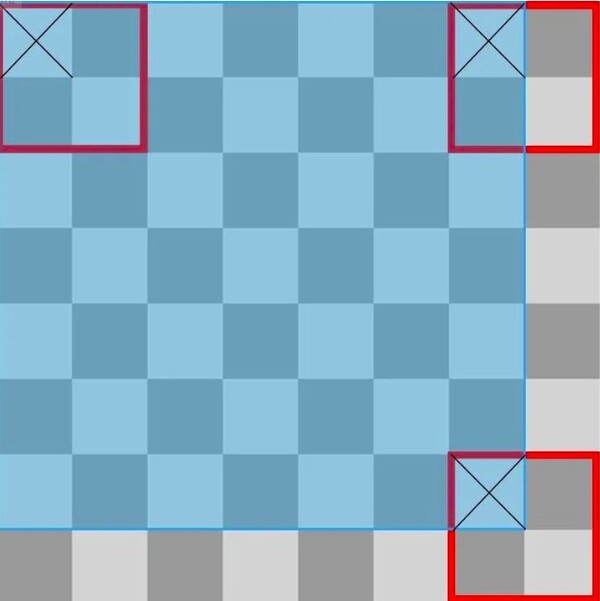
I am positive you've possibly seen a pattern by now. As we regularly make the goal square smaller by one unit, we can identify this as an ideal square number of instances. There are 2×2 starting positions a (7×7) rectangular can start from; there are 3×three beginning positions a (6×6) square can start from …

How many five × five squares Chessboard

Twenty-five small squares of a 5×5 chess board are colored with five one-of-a-kind hues to be had, such that every row contains all five available colorations, and no adjoining courts have equal shade. It is significant for the reader to remember that each square is sized the same. 
The possible colors for a 1x1 square are 100% dark; the potential colors for a 2x2 square are 50% dark and 50% light; the likely colors for a 3x3 square are 33% dark, 33% light, and 33% yellow; finally, for a 4x4 square, it would be 25%. As such, if we go by squares, then there are 25 different colors available in total!

How many 4 x 4 squares Chessboard

Therefore, we've in all = sixty four + forty nine + 36 + 25 + 16 + nine + four + 1 = 204 squares in a chessboard. Let's use the above square as an example: The first row has one square, the second row has two squares, and so on, until the last row has twenty-five squares. That equals a total of thirty-six. So our total is 204 (64+49+36+25+16+9+4+1).

How many 3 x 3 squares Chessboard

There are 64 squares on the chess board, so the total number of possible squares is 32 multiplied by itself. This means 33,554,432 possible square positions can be created with one open place with two legal moves for each moveable piece. 
A 3 x 3 grid will undoubtedly have four columns and four rows because the shaded location with the published numbers (numbers you will multiply or add collectively) is not always counted as part of the grid.

How many 2 x 2 squares Chessboard

People always wonder how many squares there are on a chessboard. It's pretty easy to find out: 
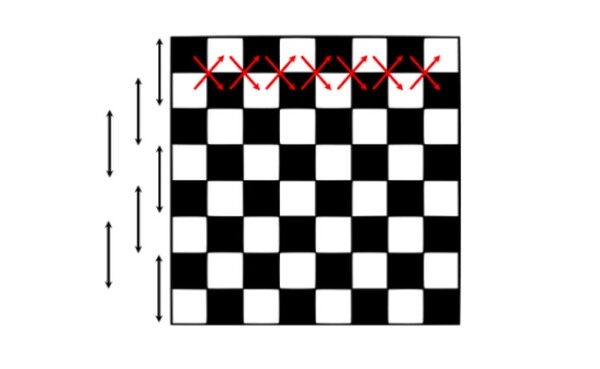
·   Start by drawing the board, including all the empty squares in the corners. There are 32 empty squares in total. Now count up how many of those spaces have one piece of chess, two pieces of chess, three pieces of chess, etc. 
With the use of what we've got to this point, we will now calculate the whole variety of squares at the chessboard: a wide variety of 1x1 squares = 8 x eight = sixty-four. Variety of 2x2 squares = 7 x 7 = forty nine. Wide variety of 3x3 squares = 6 x 6 = 36

How many 1 x 1 squares Chessboard

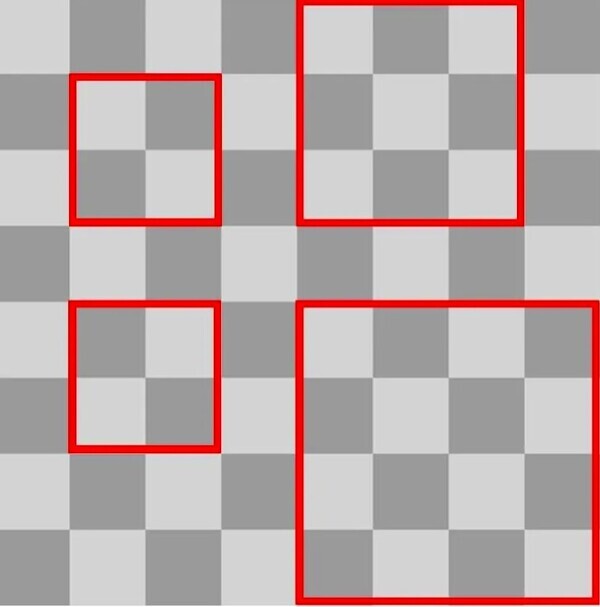
Searching closely at the chessboard, we can see that in addition to the 1 x 1 rectangular, there can be a mixture of 2 x 2, 3 x 3, 4 x 4, 5 x 5, 6 x 6, 7 x 7, and 8 x 8 squares too. To get the full quantity of squares, we need to discover all of the squares formed. 1 x 1: 8 * 8 = sixty-four squares. 2 x 2: 7 * 7 = forty nine squares.
The answer to this question is more than you might think, and it just so happens that we have all the time in the world. To find out how many squares are on a chessboard, we devised a little trick that helped us calculate the total number of squares without counting them all by hand. 
Looking at one column of chess board squares, you will notice that they all fit together like puzzle pieces.

Conclusion:

It isn't easy to know exactly how many squares are on a chessboard. We know that there is an even number of rows and columns, starting at 0, not 1. If you go through the process of counting each square from one side to the other, as well as both diagonals, you will arrive at (8 x 8 =) 64 total yards.

FAQ

Why are there 204 squares on a chessboard?

The answer is 204 squares. This is because you have to calculate how many 1 x 1 square, 2 x 2 rectangular pieces, 3 x 3 squares, and so forth are on the chessboard. These are the square numbers: 64, forty-nine, 36, 25, 16, 9, 4, 1. Those brought together equal 204.

Is a chessboard 8x8?

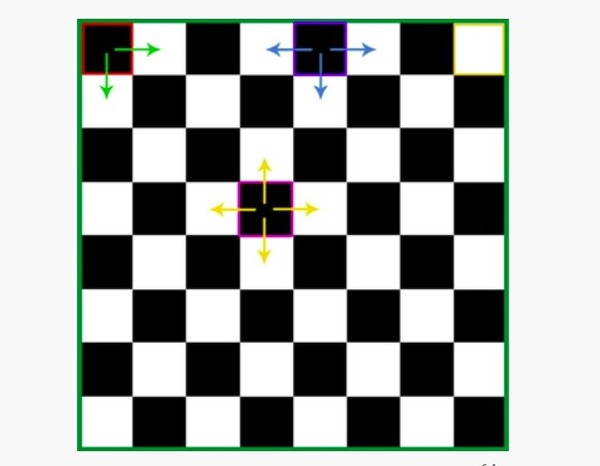
The chessboard is an 8x8 grid of alternating colored squares. Half of the sixty-four squares are called "mild squares," while the others are called "darkish squares. While putting in a chessboard, you must usually have a light square on the lower right (like the h1-square beneath the photo).

What is a square in chess called?

The vertical columns of squares, called documents, are categorized through white's left (the queenside) and right (the kingside). The horizontal rows of squares, referred to as ranks, are numbered 1 to 8, starting from White's facet of the board.

How Many Squares Are on a Chessboard?

What number of squares can you shape on a chess board? Glaringly, there may be 64 squares. Together, they form any other rectangle.